 For Daily Job Alert For Daily Job Alert |
Join Our Whats App Channel |
 For Free Study Material For Free Study Material |
Join Our Telegram Channel |
Caselet DI Tricks & Tips
In caselets, the mathematical data is represented in the form of a paragraph. Hence extracting data and establishing relationships between different data values becomes difficult. However caselets are very popular with CAT examiners.
| Positives | Negatives |
|---|---|
| 1. Most caselets seem difficult due to lack of data values, but are very easy when you get down to solving them. | 1. Data values are not easily available and hence you need to have a lot of patience to decipher a lot of it after reading the passage. |
Shortcuts to crack DI sets containing Caselets
1. Represent the data in a form such that you can easily extract the data for the questions that follow
Let us look at this CAT 2005 set to understand this.

The above set can be represented in the form of a Venn Diagram having three sets TR, FR and ER. Similarly, you can represent data in the form of a Table or a Network. The idea is to help us get the required data with a single glance upon reading the question.
2. Always start a caselet with a value that you can directly plug in.
Have a look at this CAT 1991 caselet:

The only value that one can see in the entire caselet is that at the end, he had a balance of Rs.11,000/-. This is good place to start this caselet from. Try to work out other values from this value now.
How to Solve Caselet / Paragraph DI Questions
How to solve caselets/paragraph data interpretation questions?
- Solving caselets involves a thorough understanding of the subject matter of the passage given. Read the paragraph with utmost care and analyse what the question demands. Basically be clear on what is given and what is asked.
- Try to underline all the important points in a caselet while reading it. You can always use symbols in place of names of persons, places etc to make your work a lot easier. Focus only on useful data and don’t do unnecessary approximations just to simplify the task.
- With all the important information in your hand, try to represent the data in graphical or tabular form. Represent the data in a pie chart, bar graph, table etc depending upon the feasibility and the motive of the question.
- Read the figures closely. You can use options and approximations to avoid tedious and lengthy calculations. If the question asks for relative values, there is no need for find the accurate values. Use approximation but never over-approximate.
| Bank | Shares | Real estate |
| 7.8 lakhs | 2.6 | 2.6 |
Some points to remember
- Improve your calculation speed: Caselets being calculation intensive, you have to be good with your calculations. Learn speed math techniques and practice them regularly. The more you practice the faster you become in calculations. After a considerable amount of practice, you can do the calculations in your mind. This is going to save a lot of your precious time in exam conditions. So build a intuitive number sense.
- Be thorough with the arithmetic related topics like percentages, interests, ratios and proportions as caselets are often based on these type of concepts. Otherwise you will face a lot off difficulty tackling these questions. Get the basics; understand the difference between growth and growth rate, average growth rate, cumulative average growth rate, market share by volume and market share revenue etc.
- Practice makes a man perfect. The more you practice these types of questions, the easier the questions will become. Practice questions will improve your speed and accuracy. While attempting these type of questions in exam, always try to choose the questions which will give you the answer with lesser effort, or you will end up spending a significant amount of time on lengthy calculations.
Directions: Refer to the information given below and answer the questions that follow.
There are 4 players in the cigar market of the Dominician Republic: Amergio, Byford, Christie’s and Ducatti. The sales in 1995 are of $ 100,000 (100,000 boxes of cigars) – the volumes shared by Amergio, Byford, Christie’s and Ducatti are in the ratio of 1: 2: 3: 4 and the sales is in the ratio of 4:3:2:1 respectively. The sales in 1996 show a sharp drop – both in terms of volume and in dollars. In volume terms, the demand fell by 60% and in dollar terms the figure was 50%. The shares of Amergio, Byford, Christie and Ducatti in volume terms was 2:2:3:3 and that of the ratio of prices charged was 9:6:3:1. The market picked up dramatically in 1997 – the volumes were the sum total sold in 1995 and 1996 and the sales rose by 390% over that of 1996. For 1997, sales by Christie have amounted to $84,000, which was 20% more than that made by Amergio and thrice the sales of Ducatti. The volumes share in that year was in the ratio of 1:3:4:2.
Example 1: What was the ratio of the prices charged by Amergio, Byford, Christie’s and Ducatti in 1997?
Solution: Summarising the whole information, we can deduce the following:
1. Value
For year 1995: Sales (in value) 100,000 $
Ratio of value for:
Amergio: Byford: Christie: Ducatti= 4 : 3 : 2 : 1 = 40,000 : 30,000 : 20,000 : 10,000
For year 1996: Sales (in value) = 50,000$
For year 1997: Total Sales (Value) = 50 × 4.9 = 245,000
Example 2: In which year was Sohan born?
A. Sohan at present is 25 years younger to his mother.
B. Sohan’s Sister, who was born in 1964, is 35 years younger to his mother.
Solution: From both I and II, we find that Sohan is (35 – 25) = 10 years older than his sister, who was born in 1964. So, Sohan was born in 1954.
Since, we are getting unique answer from both statements when combined, so the answer is 3rdoption.
Data Sufficiency: Key Learning
- In this article, you learned how a Data Sufficiency question is asked and what steps are to be followed in order to solve these questions systematically.
- In order to get hold on such questions you need to solve variety of questions to get exposure to different kind of Data Sufficiency questions. Read the question carefully and then solve each statement individually and combine them if required.
More Tricks To Solve Caselet DI With Example
Introduction
Caselet DI is trending these days and whenever anything starts trending, we must know about that.
Caselet DI is not new or not even out of way. It is just a mathematical form of English Comprehension. In Caselet DI, a long paragraph is given and on the basis of that, some questions are asked. Isn’t it English Comprehension?
- In a given paragraph of Caselet DI, lots of information is given.
- You have to read the paragraph carefully and then you have to note down all the key information as short as possible.
- The given information will let you draw some diagrams such as Venn diagram, tabular chart or any other diagram.
- The difference between simple DI and Caselet DI is – In simple DI, information is already given in diagrammatical forms but in Caselet DI, you have to draw a diagram on the basis of given information.
Before start solving Caselet DI, you must have knowledge of following things.
- How to draw a diagram on the basis of given information.
- Which diagram is the need of the question?
- Knowledge of Venn diagram.
- Knowledge of the relationship between fractions and their percentage forms.
- Knowledge of simplification and approximation.
- If the question belongs to CI & SI or Profit & Loss or Speed, time and distance then you must have knowledge of basic formulae of these topics.
- Must have knowledge of Ratio and Proportion.
To make few things more clear, let us take a simple question:
In a college, some students like English, some like Maths and some like Science. Some like both English and Maths but not Science, some like both Maths and Science but not English and Some like both Science and English but not Maths. Some like all three subjects.
The given information can be simply put in a Venn diagram as follow:
- A, B and C are representing the whole circle.
- A means some like English, B means some like Maths and C means some like Science.
- Region D means some like both English and Maths but not Science, region E means some like both Maths and Science but not English and region F means some like both Science and English but not Maths.
- Region G means some like all these three subjects.
After arranging the information in Venn diagram, we conclude following more information:
- Students who like only English= A- (D+F+G).
- Students who like only Maths= B-(D+E+G).
- Students who like only Science= C-(E+F+G).
NOTE: Practice more problems so that you may know the different forms of Caselet DI. While solving, must follow the above steps.
A Caselet Question Asked In BOB PO Exam, 2017
A total of 1650 employees is working in a company in different departments. The ratio of male employees to female employees in the organisation is 86:79. There are total 5 departments in the company i.e. Product Development, Sales and Marketing, R & D and Reinvestment, Finance and HR. Total 198 males work in Product Development department. 18% employees work in Sales and Marketing department in which male to female ratio is 5:4. In Finance Department, 77 males are working and the number of females in this department is 5/7th of the number of males. The number of males in Sales and Marketing department is equal to the number of females in Product Development department. The number of males in Finance department is half of the number of males in HR department. Male to female ratio in R & D and Reinvestment department is 14:19.
1. The number of males in R & D and Reinvestment department is how much more than females in Product Development?
2. Female in R & D and Reinvestment department is what % of the total number of females in the company (approximately)?
3. The number of females in Finance department is what percent less than the number of females in Product Development department?
SOLUTION:
Step 1
Step 2
- Note down the given data and make calculation as per as the requirement
- TOTAL=1650
- M:F=86:79 then we can find the number of males and females as follows:
- 86+79=165= 1650,
- 1=10,
- 86=860 (total number of male),
- 79=790 (total number of female).
- Males in Product Development department= 198
- 18% employees work in Sales and Marketing department i.e. (18/100)*1650=297;
- Male to female ratio is 5:4 in the Sales and Marketing department, so 5+4=9=297.
- Therefore, number of male in this department=5*33=165 and the number of female in this department=4*33=132.
- Number of Male in Finance department=77 and female in this department is 5/7th of male. So number of female in this department= (5/7)*77=55.
- Number of males in Sales and Marketing department is equal to the number of females in Product Development department. So number the number of female in Product Development= 165.
- Number of males in Finance department is half of the number of males in HR department. So the number of male in HR department=2*77=154.
- Male to female ratio in R & D and Reinvestment department is 14:19.
Step 3
Now put these data in the table.’
- Number of males in R & D and REINVESTMENT= 860-(198+165+77+154) = 266.
- 14=266 so 19=19*19=361 (Number of female in R & D and REINVESTMENT).
- Number of female in HR department= 790-(165+132+361+55)=77.
Step 4
Now put these data in remaining blanks in the table:
Step 5
1) Male in R & D and Reinvestment=266.
Female in Product Development=165.
So, the number of males in R & D and Reinvestment department is more than females in Product Development= 266-165=101.
2) Female in R & D and Reinvestment=361.
The total number of females=790. So, (361/790)*100=45.7%.
3) Female in Finance Department=55.
Female in Product Department=165.
So, {(165-55)/165}*100= (1/3) * 200= 33(1/3)% *2=66(2/3)% (use the concept of percentage and fraction)
Step-1 Read the given paragraph thoroughly.
Step-2 Then try to note down each and every data.
Step-3 Then deduce these data in a graphical form like tabular, Venn diagram or both.
Step-4 Now you can easily answer the questions asked.
To make it clear, I am solving a caselet problem asked in SBI PO mains.
In a medical college there are 1600 students studying Dentistry and Homeopathy. Each student from each course knows one or more languages out of English, Hindi and Bengali. 45% of the students study Dentistry and the remaining students study Homeopathy. Out of the students studying Dentistry, boys and girls are in the ratio 5:3. Out of the boys studying Dentistry, 16% know only English, 10% know only Hindi and 4% know only Bengali. 24% know English as well as Hindi, 20% know English as well as Bengali and 14% know Hindi as well as Bengali. The remaining boys know all the three languages.
Out of the girls studying Dentistry, 20% know only English, 10% know only Hindi and 10% know only Bengali. 20% know English as well as Hindi, 20% know English as well as Bengali and 10% know Hindi as well as Bengali. The remaining girls know all the three languages.
Out of the students studying Homeopathy, boys and girls are in the ratio 4:7. Out of the boys studying Homeopathy, 20% know only English, 15% know only Hindi and 5% know only Bengali. 15% know English as well as Hindi, 25% know English as well as Bengali and 10% know Hindi as well as Bengali. The remaining boys know all the three languages.
Out of the girls studying Homeopathy, 15% know only English, 15% know only Hindi and 5% know only Bengali. 20% know English as well as Hindi, 20% know English as well as Bengali and 15% know Hindi as well as Bengali. The remaining girls know all the three languages.
Questions:
2) How many students in the college know all the three languages?
3) What percent of the total number of girls in the college know Bengali?
4) How many students studying Homeopathy do not know English?
5) Out of the students studying Homeopathy, what is the ratio of the number of boys knowing English to the number of girls knowing Hindi?
Solution:
Total number of students=1600.
Number of students in Dentistry= 45% of 1600= 720.
Number of students in Homeopathy= 1600-720=880.
Ratio of boys and girls studying Dentistry= 5:3.
Number of boys studying Dentistry= (5/8) * 720= 450.
Number of girls studying Dentistry= (3/8) * 720= 270.
Ratio of boys and girls studying Homeopathy= 4:7.
Number of boys studying Homeopathy= (4/11) * 880= 320.
Number of girls studying Homeopathy= (7/11) * 880= 560.
Now make a diagram to put these values.
1) 16% of 450 + 10% of 450= 117.
20% of 270 + 10% of 270= 81.
On adding these two, we get 198.
Note: In case of either or, we use addition concept.
What are Caselets?
Caselets are paragraphs in which complete information is presented in the form of sentences. The data is in the raw form and questions are asked on its basis. Before solving questions on caselets, data has to be extracted from the paragraph. The length of the paragraph may vary from short to medium in length. Sometimes, caselets are combined with other sources of data such as tables, graphs etc.
The only disadvantage of caselets over other data interpretation questions is that we need to analyse the paragraph and the questions carefully before answering them.
3 tips to solve Data Interpretation Question Asked on Caselets
➤Read the caselets and associated questions carefully before directly jumping to solve them.
➤Arrange the data in an order to have a better understanding towards them.
➤Underline the important sentences while reading the paragraph because it will help you to draw conclusions easily.
Example: Data Interpretation Questions on caselets
A school has 400 students- boys and girls, who are in the ratio of 3:5. The students speak Hindi, English or both the languages. 12% of the boys speak only Hindi, 22% of the girls speak only English, 24% of the total students speak only Hindi and the number of boys speaking both the languages is six times the number of boys speaking only Hindi.
1) How many boys speak Hindi?
2) How many girls speak only Hindi?
3) How many students speak English?
4) The number of girls speaking only Hindi is what percent of the total number of students speaking only Hindi?
5) What is the ratio of the number of boys to the number of girls speaking both the languages
Solution:
Information analysis –
Total Number of students = 400
Ratio of boys and girls; B: G = 3: 5
Number of boys = 3/8 x 400 = 150
Number of girls = 5/8 x 400 = 250
Organise the data in a systematic order. Here, we have arranged them in a form of table.
|
Only Hindi |
Only English |
Both the languages |
|
|
Number of Boys(150) |
12% of 150 = 18 |
150 – 18 – 108 = 24 |
6 x 18 = 108 |
|
Number of Girls (250) |
96 – 18 = 78 |
22% of 250 = 55 |
250 – 78 – 55 = 117 |
|
Total number of students (400) |
24% of 400 = 96 |
Question 1: How many boys speak Hindi?
Step 1:
Solution:
In this question, we need to calculate the percentage of boys who speak Hindi. It means that we need to calculate the percentage of boys who speak only Hindi and boys who speak Hindi + English.
Step 2:
Number of boys who speak only Hindi = 18
Number of boys who speak Hindi + English = 108
18 + 108 = 126
Therefore, the number of boys who speak Hindi are 126
Question 2: How many girls speak only Hindi?
Solution:
Step 1:
In this question, we need to calculate the number of girls who speak only Hindi.
From the table above, we get to know that number of girls who speak only Hindi are 78.
Therefore, the number of girls who speak Hindi are 78.
To have an edge over data interpretation questions, practice more questions from data interpretation.
Question 3:How many students speak English?
Solution:
Step 1:
In this question, we need to calculate the number of students who speak English. So we subtract the number of students who speak only Hindi from the total number of students
Total number of students – Number of students who speak only Hindi
400 – 96 = 304
Therefore, the number of students who speak English are 304.
Question 4: The number of girls speaking only Hindi is what percent of the total number of students speaking only Hindi?
Solution:
Step 1:
Number of girls who speak Hindi – 78 [x]
Total number of students who speak only Hindi – 96 [y]
As we need to find the percentage change we know that
x/y x 100
Step 2:
78/96 x 100
81.25%
Therefore, 81.25% is the percentage change.
Question 5: What is the ratio of the number of boys to the number of girls speaking both the languages?
Solution:
Step 1:
Number of boys speaking both the languages = 108
Number of Girls speaking both the languages = 117
The ratio of the number of boys who speak both the languages: Number of girls who speak both languages
= 108: 117
Step 2:
By further simplification we get,
12:13
Therefore, the ratio of boys is to girls who speak both the languages are 12:13.
Practice Problems For Caselet DI
Example 1:
Directions for Q. 1 to 5: Refer to the following information and the answer the following questions.
People Power Corporation presently employs three Managers (A, B and C) and five recruitment agents (D, E, F, G and H). The company is planning to open a new office in San Jose to manage placement of software professionals in the US. It is planning to relocate two of the three managers and three of the five recruitment agents to the office at San Jose. As it is an organization which is
highly people oriented the management wants to ensure that the individuals who do not function well together should not be made as a part of the team going to the US.
The following information was available to the HR department of People Power Corporation.
- Managers A and C are at each others throat and therefore cannot be sent as a team to the new office.
- C and E are excellent performers in their own right. However, they do not function together as a team. They should be separated.
- D and G have had a major misunderstanding during the last office picnic. After the picnic these two have not been in speaking terms and should therefore not be sent as a team.
- D and F are competing for a promotion that is due in another 3 months. They should not be a team.
Q1. If D goes to the new office which of the following is (are) true?
I. C cannot go II. A cannot go III. H must also go
(a) I only
(b) II and III only
(c) I and III only
(d) I, II and III
2. If A is to be moved as one of the Managers, which of the following cannot be a possible working unit?
(a) ABDEH
(b) ABFGH
(c) ABEGH
(d) ABDGH
3. If C and F are moved to the new office, how many combinations are possible?
(a) 4
(b) 1
(c) 3
(d) 5
4. Given the group dynamics of the Managers and the recruitment agents, which of the following is sure to find a berth in the San Jose office?
(a) B
(b) H
(c) G
(d) E
5. If C is sent to the San Jose office which member of the staff cannot go with C?
(a) B
(b) D
(c) G
(d) F
ANSWERS: 1. (c) 2. (d) 3. (b) 4. (a) 5. (b)
Example 2
Ghosh Babu took voluntary retirement in Dec. 1991 and received a certain amount of money as retirement benefits. On Jan 1, 1992, he invested the entire amount in shares. At the end of the month, he sold all his shares and realised 25% profit. On Feb 1, he reinvested the entire amount in shares which he sold at the end of the month at a loss of 20%. Again, he invested the entire amount on Mar 1 in a new company. At the end of the month, he sold the new company to a friend and realised a profit of 20% in the process. He invested the entire amount in shares on Apr 1, which he sold at the end of the month for Rs. 1,08,000 incurring a loss of 10%.
1. What is the amount of retirement benefits received by Ghosh Babu?
a) Rs. 1,08,000
b) Rs. 1,25,000
c) Rs. 1,20,000
d) Rs. 1,00,000
2. The percentage profit received by Ghosh Babu between Jan 1 and Apr 30 is:
a) 8.00%
b) 15.00%
c) – 10.00%
d) None of these
3. The amount of loss incurred by Ghosh Babu based on his operation in Apr 1992 is:
a) Rs. 25,000
b) Rs. 12,000
c) Rs. 20,000
d) Rs. 8,000
4. The maximum amount invested by Ghosh Babu in any one month was in:
a) January
b) February
c) March
d) April
Answers:
1. d Let the amount received by Ghosh Babu in Dec. 1991 be Rs. x, as retirement benefits:
Therefore, investment in the month of Jan 1992 = 100
Profit of 25% at the end of Jan 1992.
Hence, investment in the month of Feb 1992 = 125
Loss of 20% at the end of Feb 1992
Hence, investment in the month of March 1992 = 100
Profit of 20% at the end of March 1992
Hence, investment in the month of April 1992 = 120
Loss of 10% at the end of April 1992
Therefore the amount left at the end of April 1992 = 108
Amount at the end of April 1002 = Rs. 1,08,000
Therefore, simply equating figures, he would have started with Rs 1,00,000
2. a % Profit between Jan 1 and Apr 30 = (1.08x – x/x) X 100
3. b Investment in the month of April = Rs. 1,20,000
Amount received at end of April = Rs. 1,08,000
Therefore, Loss = Rs. 12,000
4. b Maximum amount invested by Ghosh Babu is in the month of February = Rs. 1,25,000
Directions : (1-5) Study the information carefully to answer the questions that follow
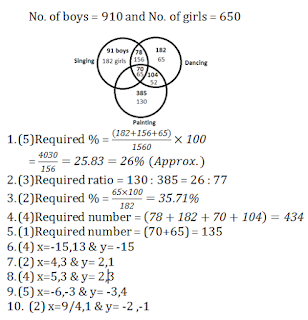
A school consisting of a total of 1560 students has boys and girls in the ratio of 7 : 5 respectively. All the students are enrolled in different types of hobby classes, viz., Singing, Dancing and Painting. One-fifth of the boys are enrolled in only Dancing classes. Twenty per cent of the girls are enrolled in only Painting classes. Ten percent of the boys are enrolled in only Singing classes. Twenty-four per cent of the girls are enrolled in both Singing and Dancing classes together. The number of girls enrolled in only Singing classes is two hundred per cent of the boys enrolled in the same. One-thirteenth of the boys are enrolled in all the three classes together. The respective ratio of boys enrolled in Dancing and Painting classes together to the girls enrolled in the same is 2 : 1 respectively. Ten per cent of the girls are enrolled in only Dancing classes whereas eight per cent of the girls are enrolled in both Dancing and Painting classes together. The remaining girls are enrolled in all the three classes together. The number of boys enrolled in Singing and Dancing classes together is fifty per cent of the number of girls enrolled in the same. The remaining boys are enrolled in only Painting classes.
1.Total number of girls enrolled in Singing is approximately what per cent of the total number of students in the school?
(1) 37
(2) 19
(3) 32
(4) 14
(5) 26
2.What is the respective ratio of the number of girls enrolled in only Painting classes to the number of boys enrolled in the same?
(1) 77 : 26
(2) 21 : 73
(3) 26 : 77
(4) 73 : 21
(5) None of these
3.Number of girls enrolled in only Dancing classes is what per cent of the boys enrolled in the same?
(1) 38.67
(2) 35.71
(3) 41.83
(4) 28.62
(5) None of these
4.What is the total number of boys who are enrolled in Dancing?
(1) 318
(2) 364
(3) 292
(4) 434
(5) None of these
5.What is the total number of students enrolled in all the three classes together?
(1) 135
(2) 164
(3) 187
(4) 142
(5) None of these
Answers with Explanation!!!!!!!!!!!
Directions (1-5): Read the information given and answer the following questions accordingly.
Not surprisingly the growth of the hotel industry is driven by the increase in the number of people using hotels and the increase in per person use of the hotel. In 2004, it is expected that there will be 200 million hotel users in India or about 20 per cent of the population will generate Rs. 50 billion in hotel revenues. Industry revenues should expand from Rs. 50 billion to Rs. 150 billion by 2008, while the number of users should grow to over 560 million or to about half the population of India in the same period.
Q1. What is the estimated population of India in 2004?
(a) 98 crore
(b) 100 crore
(c) 110 crore
(d) 115 crore
(e) None of these
Q2. What will be the simple average growth rate of population of India in the given period 2004-2008?
(a) 2%
(b) 3%
(c) 4%
(d) 4.5%
(e) None of these
Q3. What will be the growth in percentage of users in India by 2008?
(a) 100%
(b) 150%
(c) 180%
(d) 200%
(e) None of these
Q4. What will be the percentage growth of the revenues of the hotel industry in the given period?
(a) 200%
(b) 230%
(c) 260%
(d) 300%
(e) None of these
Q5. It is believed that if 50% of the population of any country can afford hotel-use, it is economically developed. Can we say that India will be a developed country by 2007?
(a) Yes
(b) No
(c) Cannot say
(d) Data inadequate
(e) None of these
Directions (6-12): Read the information given and answer the following questions accordingly.
Bihar and Orissa are the most deprived states of India. While they contain one-fifth of India’s population, they have almost one-third of India’s illiterates. In 1998, only a small fraction of Orissa and Bihar’s population was literate versus 85 per cent of Kerala’s population. More than two-thirds of the births are not attended by any medical facility, 1/10th of the infants born in Orissa and Bihar die in infancy and an equal number before reaching the age of five. Almost 90 per cent of the under five deaths are due to malnutrition.
From amongst the lucky kids who have survived for the first five years, 1/3rd of them work as child labourers and only half of the remaining are sent to school. Of those who attend classed, only 40 per cent are able to reach Std V. In India, 30 per cent of the children under 16 work as labourers. Orissa and Bihar contain 1/3rd of the child labourers in India. India has the largest population of child labourers, which is 1/15th of its total population.
In Orissa and Bihar, out of 100 children enrolled in school, 32 are girls. And out of 100 who attend Std X, only 10 are girls. Only 38 out of 100 Indian women are literate versus 57 per cent of males. Even in wealthy states such as Punjab, girls suffer from malnutrition seven times more than boys do. The total population of the country was 90 crore in 1998 and the ratio of male to female in India was 10 to 9.
Q6. According to the information provided, what percentage of the infants in Orissa and Bihar attend Std V?
(a) 11.33
(b) 10.66
(c) 13.33
(d) 12.33
(e) None of these
Q7. The number of child labourers in India in 1998 are:
(a) 15 crore
(b) 16 crore
(c) 12 crore
(d) 6 crore
(e) None of these
Q8. The Orissa and Bihar, out of 100 born, approximately how many children work as child labourers?
(a) 27
(b) 32
(c) 13
(d) 38
(e) None of these
Q9. What percentage of girl children enrolled in school reach Std X in Orissa and Bihar?
(a) 10%
(b) 32%
(c) 60%
(d) Insufficient data
(e) None of these
Q10. In 1998, the literates in Kerala exceed the literates in Orissa and Bihar by:
(a) 30%
(b) 35%
(c) 27%
(d) Insufficient data
(e) None of these
Q11. The number of literates in India in 1998 is:
(a) 16.2 crore
(b) 27 crore
(c) 43.2 crore
(d) Insufficient data
(e) None of these
Q12. The number of illiterates in Orissa and Bihar in 1998 is almost:
(a) 18 crore
(b) 13.2 crore
(c) 15.6 crore
(d) Insufficient data
(e) None of these
Directions (13-15): Read the information given and answer the following questions accordingly.
AMS, Inc. is the leader in selling ideas universe wide but its maximum revenue comes from three principal planets only, viz. Earth, Mars, Jupiter, Further, it has three products, viz. CSP, CC and CP. In a particular year, the number of units sold had a distribution as follows: The number of units of CCs sold on Mars was 12 per cent of the number of units of CPs sold on Earth. The number of units of CPs sold on Jupiter was 1000. Total number of CC units sold was 2600. Total number of CP units was 200 higher than that of the total number of units of CCs sold. The number of units of CSP sold on Mars was 10 per cent of the number of units of CP sold on Jupiter. The number of units of CSP sold on Earth was 2000. The number of units of CC sold on Earth was 15 per cent of the number of units CSP sold on Jupiter.
The prices of the units on the different planets were as follows:
Earth → Rs. 15 per unit
Mars → Rs. 10 per unit
Jupiter → Rs. 8 per unit
The number of units of CSP sold on Jupiter was 300.
The number of units of CP sold on Earth was 600.
Q13. The number of units of CC sold on Jupiter is:
(a) 1520
(b) 2483
(c) 3423
(d) 600
(e) None of these
Q14. The revenue generated on Earth is greater than that generated on Jupiter by about
(a) Rs. 8000
(b) Rs. 9000
(c) Rs. 10,000
(d) Cannot be determined
(e) None of these
Q15. The overall revenue generated is the highest from
(a) CSPs
(b) CP
(c) CCs
(d) Can’t be determined
(e) None of these
Solutions
S1. Ans.(b)
Sol. 200 million = 20% of population
⇒ Population = 200 × 5 = 1000 million = 100 crore
S2. Ans.(b)
Sol. 2004 population = 1000 million
Population in 2008 or after 4 years = 560 × 2 = 1120 million
∴ Growth rate = (120 × 100)/(1000 × 4) = (12/4)% = 3% per annum simple growth rate.
S3. Ans.(c)
Sol. Hotel users in 2004 = 200 million
Hotel users in 2008 = 560 million
∴ Growth in percentage =(560 – 200)/200=360/200=180%
S4. Ans.(a)
Sol. Total revenue in 2004 = 50 billion
Total revenue in 2008 = 150 billion
∴ Growth in percentage =(150 – 50)/50×100=100/50=200%
S5. Ans.(d)
Sol. By 2008 half or 50% of the India population will be using hotels.
We do not have any information about 2007. Hence the data is inadequate.
S6. Ans.(b)
Sol. Born 100 ⇒ 10 die at infancy
90 ⇒ 10 die till age 5
80 ⇒ 2/3×1/2×80 go to school
∴ Who attend Std. V =80×1/3×0.4=10.66%
S7. Ans.(d)
Sol. Number of child labourers in India =(90×1/15)crore = 6 crore
S8. Ans.(a)
Sol. Out of 100 born, 20 die by the age of 5.
Number of child labourers in Orissa and Bihar =1/3×80≅27
S9. Ans.(d)
Sol. Since we do not know how many children reach Std X, the answer cannot be found. Hence, option (d).
S10. Ans.(d)
Sol. Since we do not know the exact population of Kerala, Orissa and Bihar, the answer cannot be found.
S11. Ans.(c)
Sol. Number of female literates =90×9/19×0.38=16.2 crore
Number of male literates =90×10/19×0.57 = 27 crore
∴ Total literates = 16.2 + 27 = 43.2 crore
S12. Ans.(c)
Sol. Total illiterates in India = 90 – 43.2 = 46.8 crore
∴ Number of illiterates in Orissa and Bihar =1/3×46.8 = 15.6 crore
(13-15)
| Earth | Mars | Jupiter | Total | ||||||||
| Sales | Revenue | Sales | Revenue | Sales | Revenue | Sales | Revenue | ||||
| CSP | 2000 | 30,000 | 100 | 1000 | 300 | 2400 | 2400 | 33,400 | |||
| CC | 45 | 675 | 72 | 720 | 2483 | 19864 | 2600 | 21259 | |||
| CP | 600 | 9000 | 1200 | 12,000 | 1000 | 8000 | 2800 | 29,000 | |||
| Total | 39,675 | 13,720 | 30,264 | ||||||||
The answers can be read out of the table itself.
S13. Ans.(b)
Sol. 2483
S14. Ans.(e)
Sol. None of these
S15. Ans.(a)
Sol. CSP
Directions (Q. 1 – 5): Study the given information carefully to answer the questions that follow:
An organization consists of 3500 employees working in different departments, viz HR, Marketing, IT, Production and Accounts. The ratio of male to female employees in the organisation is 3 : 2. 8% of the males work in the HR department. 22% of the female work in the account department. The ratio of males to females working in the HR department is 3 : 5. One-seventh of the females work in the IT department. 46% of the males work in the Production department. The number of females is one-sixth of the males working in the same. The remaining females work in the Marketing department. The total number of employees working in the IT department is 375. 22% of the males work in the Marketing department and remaining work in the Account department.
1. The number of males working in the Account department forms approximately what per cent of the total number of males in the organisation?
(a) 6
(b) 8
(c) 10
(d) 11
(e) 12
Correct Answer :Answer: a
2. How many females work in Production department?
(a) 140
(b) 200
(c) 180
(d) 160
(e) None of these
Correct Answer :Answer: e
3. The total number of employees working in the Account department forms approximately what per cent of the total number of female employees in the organisation?
(a) 28
(b) 32
(c) 29
(d) 31
(e) 30
Correct Answer :Answer: d
4. The ratio of the numbers of females working in IT department to the numbers of males working in the same department is
(a) 15 : 8
(b) 1 : 2
(c) 8 : 15
(d) 2 : 1
(e) 7 : 11
Correct Answer :Answer: c
5. What is the total number of employees working in the Marketing and Production departments together?
(a) 1900
(b) 2040
(c) 2020
(d) 2031
(e) 2042
Correct Answer :Answer: b
- Kartik, Bhuvan and Sid entered into a partnership and invested Rs 13,000, Rs 16,000 and Rs 19,000 respectively. After 7 months, Kartik and Bhuvan added Rs 1,000 and Rs 5,000 respectively while Sid withdrew Rs 5,000. If at the end of year their annual profit is Rs 43,160, find the total profit share of Kartik and Sid.
A) Rs 28,030
B) Rs 27,190
C) Rs 26,830
D) Rs 28,420
E) Rs 27,040 - Megha, Isha and Rani entered into a partnership by investing Rs 20,000, Rs X, and Rs 22,000 respectively for 6 months, 8 months and 10 months respectively. If Isha earns a profit of Rs 16500 out of a total profit of Rs 44,550, find the total investment done by all three.
A) Rs 47,000
B) Rs 25,000
C) Rs 54,000
D) Rs 39,000
E) Rs 67,000 - Kamya, Prisha and Tisha started a business by investing Rs X, Rs (X+400) and Rs (X-200). If after the end of year, total share of profit of Kamya and Tisha is Rs 8100 out of a total profit of Rs 13,500, find the profit share of Prisha.
A) Rs 6100
B) Rs 5400
C) Rs 5100
D) Rs 6600
E) Rs 5500 - Preeti, Anu and Aarti entered into a business. Preeti invested Rs 2500 for some months, Anu invested Rs 3000 for 2 more months than Preeti and Aarti invested Rs 3500 for 3 months less than Anu. If Anu got Rs 8400, out of a total profit of Rs 19,000, then Aarti invested her money for how many months?
A) 3 months
B) 5 months
C) 4 months
D) 6 months
E) 2 months
Directions (5-7): A, B and C started a business by investing Rs 800, Rs 1600 and Rs 2000 respectively. After a quarter they invested amounts in a ratio 1 : 4 : 2. After another quarter, they invested amounts in ratio 3 : 2 : 3.In the last quarter the ratio of investments was same as in 2nd quarter. Also in the last quarter, the respective amounts of A, B and C was double than the respective amounts invested in 2nd quarter. The total investment of C before 4th quarter was Rs 1400 more than that of A during same duration. Also ratio of B’s share in profit to total profit at the end of year was 66 : 153.
- Find the total investment of A, B and C.
A) Rs 10,200
B) Rs 11,300
C) Rs 9,800
D) Rs 10,080
E) Rs 11,090 - If they respectively had invested same amounts in each quarter after quarter 1 which is equal to their respective investments in quarter 1, then what would be the profit of A at the end of year out of a total profit of Rs 19,350?
A) Rs 2510
B) Rs 3320
C) Rs 2560
D) Rs 3150
E) None of these - If the respective investments in third quarter was changed and this was in ratio 2 : 4 : 1 (other investments being the same), then what would be the total investment of all three in third quarter, if the average investment of all A B and C was Rs 3100 for whole year?
A) Rs 700
B) Rs 800
C) Rs 500
D) Rs 900
E) None of these
Directions (8-10): A, B and C started a business. They invested amounts in the ratio 1 : 3 : 2 respectively for 8 months. After this they invested amounts in ratio 2 : 3 : 4 respectively for 4 months. The average investment of A and B is Rs 2800 while average investment of B and C is Rs 3800.
- Find the total investment of C?
A) Rs 4000
B) Rs 5000
C) Rs 6000
D) Rs 4500
E) Rs 3500 - If B’s investment for both the terms (4 months and 8 months) was swapped, then find the total profit share of B and C if annual profit is Rs 46,200.
A) Rs 45,600
B) Rs 32,800
C) Rs 43,600
D) Rs 37,800
E) None of these - If A’s share in annual profit is Rs 9030, find the total profit after a year.
A) Rs 41,390
B) Rs 45,150
C) Rs 42,610
D) Rs 46,240
E) Rs 43,170










