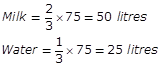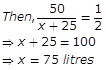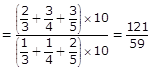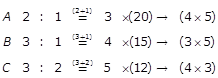Mixture and alligation Tips and Tricks

Numerical ability section is considered to be one of the toughest subjects of SSC Exams but it can be scored off well if prepared well. Mixture and alligation  is one of the toughest chapters which leaves candidates a bit confused and most of the aspirants leave these questions untouched.

To make the chapter easy for you all, we are providing you all some Basic Concept and Tricks on Mixture and alligation  which will surely make the chapter easy for you all.

Alligation 

(i) to find the mean or average value of mixture when the prices of two or more ingredients which may be mixed together and the proportion in which they mixed are given (this is Alligation Medial); and

(ii) to find the proportion in which the ingredients at given  prices must be mixed to produce a mixture at a given price. This is Alligation Alternate.

Note:

(1) The word Alligation literally means linking. The rule takes its name from the lines or links used in working out questions on mixture.

(2) Alligation method is applied for percentage value, ratio, rate, prices, speed etc and not for absolute values. That is, whenever  per cent, per hour, per kg, per km etc are being compared, we can use Alligation.

Rule of Alligation

if the gradients are mixed in a ratio, then

We represent it as under:

Then, (cheaper quantity) : (dearer quantity) = (d – m ) : (m – c)

 

Solved problems:

Ex1:  In what proportion must rice at Rs3.10 per kg be mixed with rice at Rs3.60 per kg, so that the mixture be worth Rs3.25 a kg?

Solution:

By the alligation rule :

they must be mixed in the ratio 7 :3.

Milk and Water

Ex2: A mixture of certain quantity of milk with 16 liters of water is worth 90 P per liter. If pure milk be worth Rs1.08 per liter, how much milk is there in the mixture?

Solution:

The mean value is 90 P and price of water is 0 P.

By the Alligation Rule, milk and water are in the ratio of 5 :1.

quantity of milk in the mixture = 5 ×16 = 80 liters.

Ex3: 300 gm of sugar solution has 40% sugar in it. How much sugar should be added to make it 50% in the solution?

Solution:

The existing solution has 40% sugar, and sugar is to be mixed ; so the other solution has 100% sugar. So, by alligation method;

the two mixture should be added in the ratio 5 :1

Therefore, required sugar =

Direct formula :

Quantity of sugar added =

In this case,

Ans =

 

5 Most Important Questions with Short Tricks on Mixture & Alligation

Question 1: In a mixture of 75 litres, the ratio of milk to water is 2 : 1. The amount of water to be further added to the mixture so as to make the ratio of the milk to water 1 : 2 will be

(1) 45 litres

(2)  60 litres

(3)  75 litres

(4)  80 litres

Solution:

In 75 litres of the mixture.

Let x litres of water be added,

Short Trick:

3 units = 75 l because 75 l was in ratio 2 : 1 initially.

Question 2: A mixture of 40 litres of milk and water contains 10% water. How much water must be added to make 20% water in the new mixture?

(1)  3 litres

(2)  4 litres

(3)  5 litres

(4)  6 litres

Solution 

(8 x 5 = 40 l which is the initial quantity. Hence 1 is also multiplied by 5)

Short Trick:

(40 + x) x 20% = 4 + x

x = 5l

Question 3: In three vessels each of 10 l capacity, mixture of milk and water is filled, the ratios of milk and water are 2 : 1, 3 : 1 and 3 : 2 in the three respective vessels, if all the three vessels are emptied into a single large vessel, find the proportion of milk and water in the mixture.

(1)  121 : 59

(2)  123 : 59

(3)  125 : 59

(4)  127 : 59

Solution:

2^nd Method :

L.C.M. of 3, 4 & 5. Hence 3 is multiplied by 4 x 5; 4 is multiplied by 3 x 5; 5 is multiplied by 3 x 4.

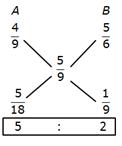
Question 4: Vessels A and B contain mixtures of milk and water in the ratios 4 : 5 and 5 : 1 respectively. In what ratio should quantities of mixture be taken from A and B to form a mixture in which milk and water is in the ratio 5 : 4?

(1)  2 : 5

(2)  4 : 3

(3)  5 : 2

(4)  2 : 3

Solution: 

Quantity of milk in vessel A

Quantity of milk in vessel B

Quantity of milk in final mixture

So, mixture of vessels A and B are mixed in ratio of 5 : 2.

2^nd Method :

Question 5: 50 g of an alloy of gold and silver contains 80% gold (by weight). The quantity of gold, that is to be mixed up with this alloy, so that it may contain 95% gold, is

(1)  200 g

(2)  150 g

(3)  50 g

(4)  10 g

Solution: 

Quantity of gold in the alloy

Let x gm of gold is added, then

Other Method: 

1 unit when multiplied by 50 gives 50g. Hence 3 unit is also multiplied by 50.