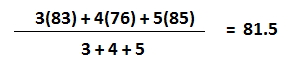Mixtures & Alligations Tricks & Tips
“One Topic, to rule them all”
Alligation is a method of solving arithmetic problems related to mixtures of ingredients. Please note that alligation method is applied for percentage value, ratio, rate, prices, speed, etc. and not for absolute value. That is whenever per cent, per km, per hour, per kg, are being compared, we can use Alligation.
Common trick for Ratio-Proportion and Mixture Alligation : Almost 50% of the questions are solvable just by going through the options. Just go through the questions I have solved in this article and you will know the approach.
Rule of Alligation
if the gradients are mixed in a ratio, then
![]()
We represent it as under:

Then, (cheaper quantity) : (dearer quantity) = (d – m ) : (m – c)
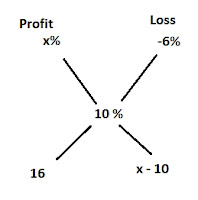
Q.1
Given SP = Rs. 320/kg, Profit = 20%
Hence CP = 320/1.2 = Rs. 800/3
So the Mean price is Rs. 800/3 per kg
Now you can apply the formula-
Type 1 : Type 2 = 280 – 800/3 : 800/3 – 180 = 2 : 13
Answer : (B)
Both the containers have equal capacity. Let us assume that both containers are of 28 litres. Why 28? Because 28 is the LCM of (3 + 1) and (5 + 2) or 4 and 7. So taking the capacity as 28 litres will make your calculations easier.
In Container 1, we have (3/4)*28 = 21 litres of milk and (1/4)*28 = 7 litres of water.
In Container 1, we have (5/7)*28 = 20 litres of milk and (2/7)*28 = 8 litres of water.
Total milk in both the containers = 21 + 20 = 41
Total water in both the containers = 7 + 8 = 15
Milk : Water = 41 : 15
Answer : (D)
Shortcut
Container 1 has 3 times more milk than water
Container 2 has 2.5 times more milk than water
When the contents of the two containers are mixed, the milk will still be more than water. How much more ? Somewhere between 2.5 and 3 times
(D) is the only option where the quantity of milk is around 2.7 times (i.e. between 2.5 and 3) that of the water.
Milk in vessel A = 4/7
Milk in vessel B = 2/5
Milk in vessel C = 1/2 (because in vessel C, milk and water are present in 1:1 ratio)
You have to mix 4/7 and 2/5, to produce 1/2. Hence 1/2 is the Mean Price.
A : B = (1/2 – 2/5)/(4/7 – 1/2) = 14 : 10 = 7 : 5
Shortcut
Final ratio of the three varieties is 5 : 7 : 9
The question asks us the quantity of third variety of tea in the final mixture. From the above ratio, it is clear that the quantity of the third variety is a multiple of 9. So 45 is the only option possible.
Answer : (D)
Method
Let the three quantities be 4x, 5x and 8x
New quantities are 4x + 5, 5x + 10 and 8x + p
Now 4x + 5 : 5x + 10 : 8x + p = 5 : 7 : 9
(4x + 5)/(5x + 10) = 5/7 and (4x + 5)/(8x + p) = 5/9
Solving 1st equation, we get x = 5
Solving 2nd equation, we get p = 5
In the final mixture the quantity of the third variety is 8x + p = 8*5 + 5 = 45
In this question we will use the below formula
So from the above formula
(Quantity of acid left)/(Quantity of acid in the original mixture) = (1 – 4/20)^2 = 16:25
Answer : (A)
Let the original quantities of A and B be 4x and x
In 10 litres, quantity of A = 4/5 * 10 = 8 litres
In 10 litres, quantity of B = (10 – 8) = 2 litres
New quantities of A and B are 2x and 3x
(Original Quantity of A) – (New quantity of A) = 8 litres[Because after taking out 10 litres of the mixture, the quantity of liquid A reduced by 8 litres]
So, 4x – 2x = 8
or x = 4
Hence quantity of liquid A in original mixture = 4*4 = 16 litres
Answer : (C)
Note : In the above question, there were two different ratios 4:1 and 2:3, then too I took the same constant of proportionality for them, i.e. ‘x’ because the following two conditions were met:
1. The volume of mixture did not change (Like in this question 10 litres were replaced, not removed)
2. The two ratios had same no. of parts (4:1 and 2:3 both have 5 parts)
You can take different constant to solve the question, but that will make the calculations little lengthy.
Since the ratio of alcohol and water is 1:4, hence quantities of alcohol and water in the mixture are 3 litres and 12 litres respectively.
Total volume will become 18 litres after adding 3 litres water
% of alcohol = 3/18 * 100 = 50/3%
Answer : (B)
Q. 8)
Let us solve some CGL questions that may not seem to belong to Mixture-Alligation category, but are easier to solve via Alligation formula…
Alternate method
This method is equally easy.
Let the distance travelled on foot be X km. Then distance travelled on bicycle = (61 – X)
Now he travelled for 9 hours. Hence
X/4 + (61 – X)/9 = 9
Solve for X
You will get X = 16 km
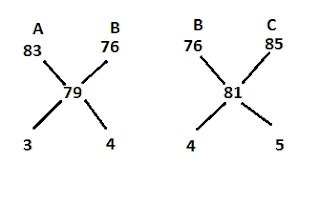
A : B = 3 : 4 and B: C = 4 : 5
A : B : C = 3 : 4 : 5
Average score of classes A, B and C =
Practice Sets On Mixtures & Alligations
- An alloy contains iron, copper and zinc in the ratio of 3:4:2. Another alloy contains copper, zinc and tin in the ratio of 10:5:3. If equal quantities of both alloys are melted, then weight of tin per kg in the new alloy
a) 1/8 kg
b) 1/10 kg
c) 1/12 kg
d) 1/14 kg
e) None of these - 8 litres are drawn from a flask containing milk and then filled with water. The operation is performed 3 more times. The ratio of the quantity of milk left and total solution is 81/625. How much milk the flask initially holds?
a) 10ltr
b) 20ltr
c) 30ltr
d) 40ltr
e) None of these - A 40 litre mixture contains milk and water in the ratio of 3:2. 20 litres of the mixture is drawn of and filled with pure milk. This operation is repeated one more time. At the end what is the ratio of milk and water in the resulting mixture?
a) 5:1
b) 6:1
c) 8:1
d) 9:1
e) None of these - Two vessels contain milk and water in the ratio of 7:3 and 2:3 respectively. Find the ratio in which the contents of both the vessels must be mixed to get a new mixture containing milk and water in the ratio 3:2.
a) 2:1
b) 2:3
c) 3:1
d) 3:5
e) None of these - How many Kgs of rice A costing rupees 20 per kg must be mixed with 20 kg of rice B costing rupees 32 per kg, so that after selling them at 35 rupees per kg, he gets a profit of 25%.
a) 10 kg
b) 40 kg
c) 24 kg
d) 25 kg
e) None of these - How many litres of water must be added to 60 litre mixture that contains milk and water in the 7:3 such that the resulting mixture has 50% water in it?
a) 12
b) 16
c) 24
d) 28
e) None of these - A sample of x litre is replaced from a container containing milk and water in the ratio 2:3 by pure milk. If the container hold 30 litres of the mixture, and after the operation proportion of milk and water is same. Find the value of X?
a) 4
b) 5
c) 6
d) 7
e) None of these - Two cans P and Q contains milk and water in the ratio of 3:2 and 7:3 respectively. The ratio in which these two cans be mixed so as to get a new mixture containing milk and water in the ratio 7:4.
a) 4:7
b) 7:3
c) 7:4
d) 7:5
e) None of these - A trader mixes 6ltr of milk costing 5000 rupees with 7ltr of milk costing 6000 rupees per litre. The trader also mixes some quantity of water to the mixture so as to bring the price to 4800 per litre. How many litres of water is added
a) 1ltr
b) 2ltr
c) 3ltr
d) 4ltr
e) None of these - How many kilograms of rice costing Rs. 9 per kg must be mixed with 27kg of rice costing Rs.7 per kg so that there may be gain of 10% by selling the mixture at Rs.9.24 per kg?
a) 63
b) 56
c) 49
d) 35
e) None of these
- A man buys milk at the rate of 5 rupees per litre and mixes it with water. By selling the mixture at Rs 4 a litre he gains 25 percent. How much water did each litre of the mixture contain?
a) 9/25
b) 11/25
c) 16/25
d) 19/25
e) None of these - A mixture containing milk and water in the ratio 3:2 and another mixture contains them in the ratio 4:5. How many litres of the later must be mixed with 3 litres of the former so that the resulting mixture may contain equal quantities of milk and water?
a) 3.3/4 litre
b) 4.1/2 litre
c) 5.2/3 litre
d) 5.2/5 litre
e) None of these - Two vessels contain milk and water in the ratio of 7:3 and 2:3 respectively. Find the ratio in which the contents of both the vessels must be mixed to get a new mixture containing milk and water in the ratio 3:2.
a) 2:1
b) 2:3
c) 3:1
d) 3:5
e) None of these - In 80 litre mixture of milk and water, water content is 40 percent. The trader gives 20 litre of the mixture to the customer and adds 20 litres of water to the mixture. What is the final ratio of milk and water in the mixture?
a) 9:13
b) 9:11
c) 11:9
d) 12:7
e) None of these - 70 litres of a mixture of milk and water contains 20% water. How much water should be added so that the mixture has 28% water?
a) 50/9 litre
b) 60/9 litre
c) 70/9 litre
d) 100/9 litre
e) None of these - Rice worth Rs. 110 per kg and Rs. 95 per kg are mixed with a third variety in the ratio 1:1:2. If the mixture is worth Rs. 115 per kg, the price of the third variety per kg will be
a) 117.5
b) 127.5
c) 137.5
d) 147.5
e) None of these - A trader has 60 kg of pulses, one part of which is sold at 8% profit and the rest is sold at 14% profit. He gains 12% on whole. What is the quantity sold at 14% profit?
a) 20kg
b) 30kg
c) 40kg
d) 50kg
e) None of these - Two cans of 60 and 80 litres are filled with the mixtures of milk and water. The proportion of milk and water in the cans being 5:7 and 9:7 respectively. If the contents of the two cans are mixed and 30 litres of the water is added to the whole, then find the ratio of milk and water in the final mixture?
a) 7:9
b) 7:10
c) 8:11
d) 9:7
e) None of these - There are three vessels each of 20 litre capacity is filled with the mixture of milk and water. The ratio of milk and water are 2:3, 3:4 and 4:5 respectively. All the vessels are emptied into fourth vessel, then find the ratio of milk and water in the final mixture.
a) 401/543
b) 401/544
c) 401/545
d) 401/546
e) None of these - In two alloys copper and zinc are in the ratio of 1:4 and 3:1 respectively. 20 kg of first alloy and 32 kg of second alloy and some pure zinc are melted together. An alloy is obtained in which the ratio of copper and zinc was 3:5. Find the quantity of zinc melted.
a) 65/3 kg
b) 67/3 kg
c) 68/3 kg
d) 71/3 kg
e) None of these
- In two alloys copper and zinc are in the ratio of 1:3 and 4:1 respectively. 20 kg of first alloy and 35 kg of second alloy and some quantity of pure zinc is melted together. The final alloy has copper and zinc in the ratio of 5:4. Find the amount of pure zinc melted.
a) 4.2
b) 4.4
c) 4.6
d) 4.8
e) None of these - In what ratio three kinds of rice costing 1.45rs, 1.54rs and 1.70rs must be mixed so that the mixture can be sold at 1.65rs per kg.
a) 11:21:44
b) 20:11:44
c) 11:20:44
d) 44:20:11
e) None of these - A container filled with liquid containing 4 parts of water and 6 parts of milk. How much of mixture must be drawn off and filled with water so that the mixture contains half milk and half water.
a) 1/4
b) 1/3
c) 1/6
d) 1/5
e) None of these - A trader has 1500 kg of wheat. One part of it is sold at 10 percent profit and other part at 18 percent profit. He gains a total of 16 percent on the whole lot. The quantity sold at 10% is-
a) 275
b) 375
c) 475
d) 575
e) None of these - Two cans P and Q contains milk and water in the ratio of 3:2 and 7:3 respectively. The ratio in which these two cans be mixed so as to get a new mixture containing milk and water in the ratio 7:4.
a) 4:7
b) 7:3
c) 7:4
d) 7:5
e) None of these - A dishonest seller professes to sell his milk at cost price but he mixes water with milk and gains 25 percent, then find the percentage of milk in the mixture.
a) 60%
b) 70%
c) 80%
d) 90%
e) None of these - Fresh fruit contains 75 percent water and dry fruit contains 20 percent water. How much dry fruit can be obtained from 150 kg of fresh fruit.
a) 45
b) 46
c) 47
d) 48
e) None of these - How much water must be added to 50 litre of milk costing 10 rupees per litre so as to bring the cost of milk to 8 rupees per litre.
a) 11.5
b) 12.5
c) 13.5
d) 14.5
e) None of these - A trader mixes 6ltr of milk costing 5000 rupees with 7ltr of milk costing 6000 rupees per litre. The trader also mixes some quantity of water to the mixture so as to bring the price to 4800 per litre. How many litres of water is added
a) 1ltr
b) 2ltr
c) 3ltr
d) 4ltr
e) None of these - There are three vessels each of 20 litre capacity is filled with the mixture of milk and water. The ratio of milk and water are 2:3, 3:4 and 4:5 respectively. All the vessels are emptied into fourth vessel, then find the ratio of milk and water in the final mixture.
a) 401/543
b) 401/544
c) 401/545
d) 401/546
e) None of these