Mixtures & Alligations Tips & Tricks
INTRODUCTION TO MIXTURE AND ALLIGATION:
Hi Aspirants today we are going to discuss on a topic Mixture and Alligation. This topic carries 1 mark in prelims and 2 mark in mains exam.This topic can be implemented in Topics such as Time and Distance ,Ratio and Proportions.
MIXTURE:
When two or more components are mixed together then it is known as Mixture
Mixture is of two types they are:
1.Simple Mixture:When two or more different ingredients are mixed together,a simple mixture is formed
2.Compound Mixture:When two or more simple mixtures are mixed together, a compound mixture is formed.
ALLIGATION:
It enables us to find the ratio in which two or more ingredients at the given price must be mixed to produce a mixture of desired price.
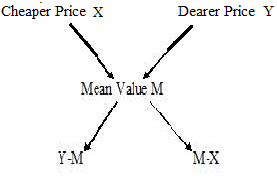
Where M=Mean Value which is between X and Y, X=Cheaper Price, Y=Price higher than the Mean Value
#.1.TYPE 1:
MIXTURE CONTAINING TWO OR MORE INGREDIENTS:
1.A Shopkeeper Sells Rice Of 1kg For Rs.75 W Two Quality Of Rice Whose Worth For 1kg Is Rs.70 And Rs.80.
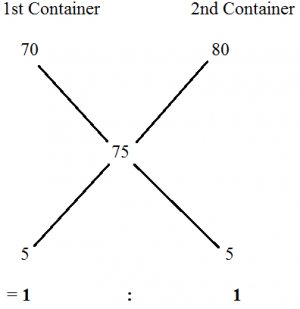
Therefore for 1kg of rice the two quality of rice should be mixed in the ratio 1:1
TYPE 2:
BASED ON PROFIT OR LOSS:
1.A Dishonest Milkman Professes To Sell His Milk At Cost Price But He Mixes It With Water And Thereby Gains 25%.The Percentage Of Water In The Mixture?
Let the Cost Price of Pure Milk be Rs.1,
Selling Price of (Milk+ Water) is also Rs.1. which is 25% more than the Costprice
Therefore CostPrice = (100/125)*1=(4/5)
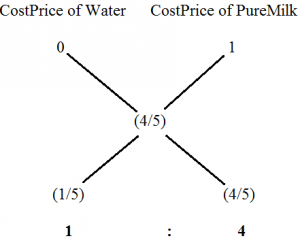
The Percentage of water mixed in the mixture =*100=20%
2.The Ratio ,In Which Tea Costing Rs.192 Per Kg Is To Be Mixed With Tea Costing Rs.150 Per Kg So That The Mixed Tea When Sold For Rs.194.40 Per Kg,Gives A Profit Of 20%.
| Quality | Cost Price | Selling Price | Selling Price after Profit/Loss |
| 1st Quality | 150 | 194.40 after 20% profit | |
| 2nd Quality | 192 |
Finding Selling Price after Giving Profit over Cost Price:
Let the Cost Price be X
Selling Price=X*(120/100) = 194.40
X=(19440/120) ⇒ X=162
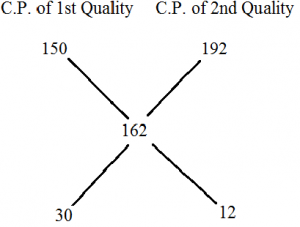
30:12 ⇒ 5:2
The 1st and 2nd quality of rice should be mixed in the ratio:5:2
Remember:
If there is a loss in the transaction indicate it by the ‘-‘ negative sign before the number.
TYPE 1:
BASED ON THREE INGREDIENTS OR THREE RATIO’S:
1.The Grocer sells apples of 3 varieties whose price per kg’s are Rs.40,Rs.60 and Rs. 90 .If he sells 1kg of apple by missing all these 3 varieties for Rs.80 what is the ratio by which these varieties are to be mixed?
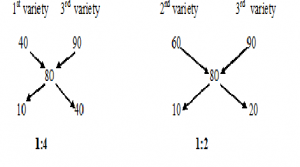
| 1st variety | 3rd variety | 2nd variety
|
| 1 | 4 | |
| 2 | 1 |
Ratio’s by which these 3 Qualities are missed are=1:2:4
2.Tea worth Rs.126 per kg and Rs.135 per kg are mixed with a third variety in the ratio 1:1:2.If the mixture is worth Rs.153 per kg, the price of the third variety per kg will be?
Given that the tea worth Rs.126 and Rs.135 per kg is mixed in the ratio 1:1.
Therefore price for 1kg= = Rs.130.50
Now we have two price 130.50 and the price of the third variety is X
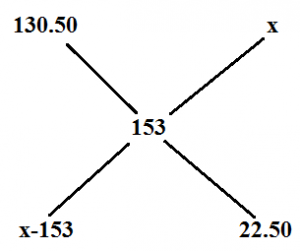
The price of the third variety=Rs.175.50
3.There are three vessels of equal capacity holds milk and water in the ratio of 1:2,2:3 and 1:4if the content of all the three vessel s are mixed in asingle vessel then.Find the ratio of milk and water in the new vessel?
Here there are three ratio’s
1:2=3Part
2:3=5Part
1:4=5Part
Lcm =15Litres
| Milk | Water |
| 5Litres | 10Litre |
| 6Litre | 9Litre |
| 3Litre | 12Litre |
| 14Litres | 31Litre |
The new Ratio is 14:31
TYPE 2:
BASED ON REMOVING AND ADDING SOME QUANTITY :
1)How much water must be added to 60 litres of milk at 1.5 litre for Rs.20,So as to have a mixture worth Rs.10 2/3 per litre?
C.P for 1.5 litre of milk =Rs.20
C.P for 1 litre of milk=Rs.40/3
C.P for 1 litre of water=Rs.0
Given Mean value=Rs.32/3
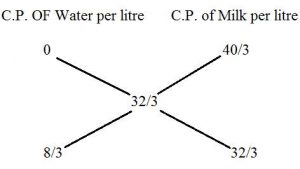
8/3 : 32/3= 1:4
Therefore water in the mixture in 60 litres is (1/5)*60=12 litres
2)In a 729 litres mixture of milk and water ,the ratio of milk to water is 7:2.To get a new mixture containing milk and water in the ratio 7:3,the amount of water to be added is?
| 729 | |
| 7x | 2x |
9x=729 x=81
Old Mixture New Mixture
7:2 7:3
Already the mixture contains water in the proportion 2x and the new mixture contains water in the proportion 3x .So x quantity of water is added to the mixture .Therefore 81 litres of water is added to the new mixture.
3)8 litres are drawn from a cask filled with wine and is then filled with water .This operation is performed three more times.The ratio of the quantity of wine now left in the cask to that of the total solution is 16:81.How much wine does the cask originally ?
When the final amount of solute that is not replaced calculated as:
Initial Amount * (Volume After Removal/Volume After Replacing)^n
Final ratio of solute not replaced to total is
Initial Ratio * (Volume After Removal/Volume After Replacing)^n
Here let us assume the initial value is 1 then Wine .Let the Quantity of wine in the cask originally be x
Therefore
1* [(x-8)^4/x]
=[16/81]
x=24litres
Wine originally in the cask is 24 Litres
4)A vessel contains mixture of liquids A and B in the ratio 3:2.When 20 litres of the mixture is taken out and replaced by 20litres of liquid B,the ratio changes to 1:4.How many litres of liquid A was there initially present in the vessel?
Original ratio=3:2
3/5 * (5X-20)/5x=1/4
X=6 then the liquid A in the vessel =3X=3*6=18Litres
IMPORTANCE OF MIXTURE AND ALLIGATION:
Mixture and Alligation is used in other Miscellaneous Topic such as
Ratio and Proportion
Time and Distance
You can reduce the time taken to solve the problem if you use Mixture and Alligation Method
#.1.TYPE 1:
BASED ON ALLOYS:
1.A milk vendor has 2 cans of milk. The first contains 25% water and he rest milk.The second container 50% water.How much milk should he mix from each of the containers so as to get 12 litres of milk such that the ratio of water to milk is 3:5?
Given that
Milk in 1st Can = 3/4
Milk in 2nd Can =1/2
We want milk in the ratio=5/8
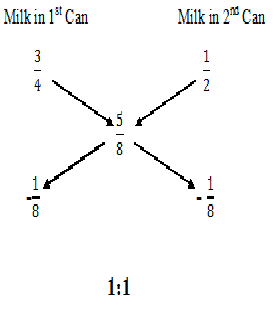
(1/2)*12=6litres So, quantity of mixture taken from each can=6litre
2.The milk and water in two vessels A and B are in the ratio 4:3 and 2:3 respectively .In what ratio the liquids in both vessels be mixed to obtain a new mixture in vessel c consisting half milk and half water?
Mean Value=(1/2)
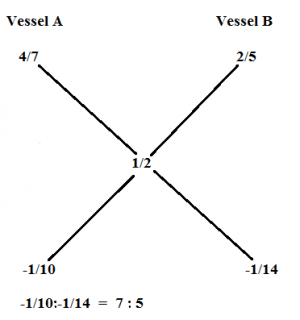
Therefore Milk and Water should be mixed in the Ratio of 7:5
#.2 TYPE 2:
FINDING NUMBER OF PERSONS :
1.In a zoo there are Rabbits and Pigeons.If heads are counted ,there are 200 and if legs are counted there are 580.How many pigeons are there?
Total heads Count=200
Total Legs Count=580
Average Leg Count =580/200
= 29/10
Average leg Count for Rabbit =4,
Average leg Count for Pigeons=2
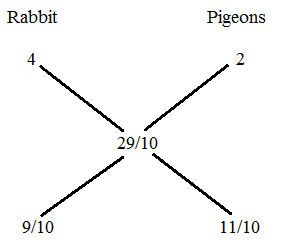
9:11
Thus the number of Pigeons is * 200=110 pigeons
2.The total number of people in the village is 5000.If the male and female increases by 10% and 15% respectively,the population of the village becomes 5600.What is the number of male in the village?
Total number of people in the village=5000
10% of the village =500 15% of the village=750
Increased number of people=5600-5000=600
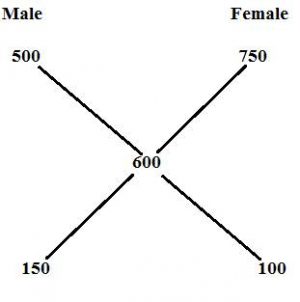
=150:100
=3:2
Male in village=3000 ⇒ (5000*3/5=3000)
#.3. TYPE 3:
PARTIAL WORK:
1.A shopkeeper sells 1000kg of sugar ,part of which he sells at 8% profit and the rest at 18% profit .He gains 14% on the whole. The quantity sold at Rs.18% is?
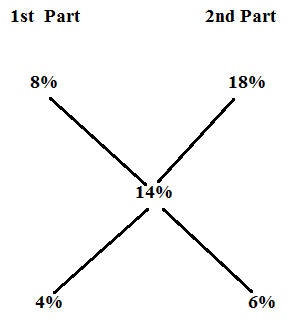
4:6
=2:3
Therefore the 1000kg sugar is sold in the ratio 400:600
Sugar sold at the profit of 18%=600kg
1)BASED ON FINDING INDIVIDUAL CAPACITY
2)BASED ON DIFFERENT CAPACITY
3)BASED ON RATIO’S
1)A Jar Contains A Mixture Of Milk & Water In The Ratio 4:1.When 10 Litres Of The Mixture Is Taken Out & 10 Litres Of Water Is Poured Into The Jar,The Ratio Becomes 2:3.How Many Litres Of Milk Was In The Jar?
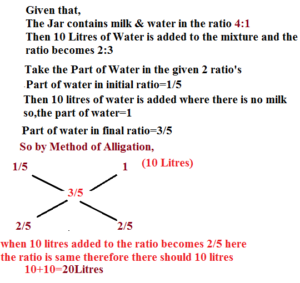
PRACTICE THIS SUM:
1)A Vessel Contains mixture of liquids A and B in the ratio 3:2.When 20 litres of the mixture is taen out and replaced by 20 litres of liquid B,the ratio changes to 1:4.How many litres of liquid A was there initially present in the vessel?
2)A can contains a mixture of two liquids in proportion 7:5.When 9 litres of mixture are drawn off and the can is filled with B,the proportion of A and B becomes 7:9.How many litres of liquid A was contained by the can initially?
#.2.TYPE 2:
BASED ON DIFFERENT CAPACITY:
1)Three glasses of Sizes 5 litres,10 litres & 15 litres contain mixture of Milk & Water in the ratio 3:2,4:6&4:11 respectively.The Contents of all the three glasses are poured into single vessel.Find the ratio of Milk to Water in the resultant mixture.
To find the Ratio of Milk to Water in the resultant Mixture,
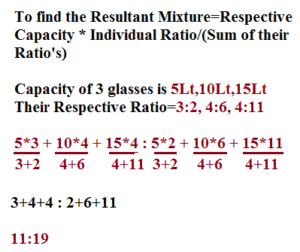

PRACTICE THIS SUM:
1)Three bottle of Capacity 6 Litres,12 Litres and 4 litres contain mixture of Sugar water and honey in the ratio 1:5 , 7:5 & 1:3 respectively.The contents of all the three bottles are poured into single bottle.Find the ratio of Sugar water to honey in the resultant mixture?
2)Four glasses of sizes 2Litres,8 Litres,12 Litres & 16 Litres contain mixture of milk & Water in the ratio 1:1 ,3:5,7:5,9:7 respectively.The Contents of all the four glasses are poured into single glass.Find the ratio of Milk & Water in the resultant Mixture?
#.3. TYPE 3:
BASED ON RATIO’S:
1)In an Alloy,Zinc and Copper are in the ratio 3:4,In the Second alloy the same elements are mixed in the ratio 7:2.In what ratio should these two alloys be mixed to form a new alloy in which the two elements are in the ratio 5:6?
The ratio is written as
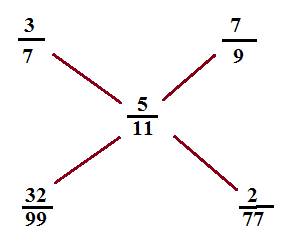
The ratio is 32/99:2/77
PRACTICE THIS SUM:
1)In An Alloy The Zinc & Copper Are In The Ratio 1:3.In The Second Alloy The Same Elements Are In The Ratio 4:3.In What Ratio Should These Two Alloys Be Mixed To Form A New Alloy In Which The Two Elements Are In The Ratio 4:5?
2)A jar full of lime juice contains juice and sugar water in the ratio 2:3.In the 2nd Jar the same elements are in the ratio 7:2.In what ratio should these two elements be mixed to for a new alloy in which the two elements are in the ratio 6:5?
Practice Sets On Mixtures & Alligations
- Vikram covered 180 km distance in 10 hours. The first part of his journey he covered by Car, then he hired a Rickshaw. The speed of the car and rickshaw is 25 kmph and 15 kmph respectively. The ratio of the distances covered by the car and the rickshaw is
A. 7:9
B. 7:3
C. 7:5
D. 7:2
E. 7:4 - A mixture of wheat is sold at Rs.3 per Kg. This mixture is formed by mixing the Wheat of Rs.2.10 per kg and Rs.2.52 per kg. What is the ratio of price of cheaper to the costlier quality in the mixture if the profit of 25% is being earned
A.2:5
B.2:1
C.2:3
D.2:7
E.2:9 - From the 50 liters of a chemical solution, 5 liters of chemical solution is taken out and after it, 5 liters of water is added to the rest amount of chemical solution. Again 5 liters of chemical solution and water is drawn out and it was replaced by 5 liters of water. If this process is continued similarly for the third time, the amount of chemical solution left after the third replacement
A. 32.75L
B. 30.80L
C. 36.45L
D. 30.25L
E. 38.50L - From a container of milk, which contains 200 liters of milk, the seller replaces each time with water when he sells 40 liters of milk(or mixture). Every time he sells out only 40 liters of milk(or mixture). After replacing the milk with water 4th time, the total amount of water in the mixture is
A. 75.82L
B. 81.92L
C. 85.28L
D. 87.45L
E.None of these - A jar was full with Milk. A person used to draw out 20% of the Milk from the jar and replaced it with water. He has repeated the same process 4 times and thus there was only 512 gm of milk left in the jar, the rest part of the jar was filled with the water. The initial amount of milk in the jar was:
A. 1.50 kg
B. 1.30 kg
C. 1.40 kg
D. 1.25 kg
E. 1.75 kg - From a container of Milk, a thief has stolen 15 liters of milk and replaced it with same quantity of water. He again repeated the same process. Thus in three attempts, the ratio of Milk and water became 343:169. The initial amount of Milk in the container was:
A.140 litre
B.130 litre
C.125 litre
D.120 litre
E.115 litre - The ratio of Solution “A” and Solution “B” in the container is 3:2 when 10 liters of the mixture is taken out and is replaced by the Solution “B”, the ratio become 2:3. The total quantity of the mixture in the container is:
A.25L
B.20L
C.30L
D.45L
E.None of these - From a container, 6 liters Solution “A” was drawn out and was replaced by water. Again 6 liters of the mixture was drawn out and was replaced by the water. Thus the quantity of Solution “A” and water in the container after these two operations is 9:16. The quantity of the mixture is:
A.35L
B.25L
C.20L
D.15L
E.10L - The diluted Milk contains only 8 liters of Milk and the rest is water. A new mixture whose concentration is 30%, is to be formed by replacing Milk. How many liters of the mixture shall be replaced with pure Milk if there was initially 32 liters of water in the mixture?
A. 3 litre
B. 4 litre
C. 8 litre
D. 5 litre
E. None of these - In a school, the average weight of boys in a class is 30 kg and the average weight of girls in the same class is 20kg. If the average weight of the whole class is 23.25 kg, what could be the possible strength of boys and girls respectively in the same class?
A. 18 and 19
B. 13 and 27
C. 16 and 15
D. 15 and 13
E.None of these
- A vessel is filled with liquid, 4 parts of which are water and 5 parts syrup. How much of the mixture must be drawn off and replaced with water so that the mixture may be half water and half syrup?
A.1/9
B.1/10
C.1/5
D.1/12
E.1/7 - Rice worth Rs. 126 per kg and Rs. 134 per kg are mixed with a third variety in the ratio 1 : 1 : 2. If the mixture is worth Rs. 177 per kg, the price of the third variety per kg will be:
A.254
B.216
C.224
D.238
E.262 - One quantity of rice priced at Rs 9.30 per Kg is mixed with another quality at a certain rate in the ratio 8:7. If the mixture so formed be worth Rs 10 per Kg, what is the rate per Kg of the second quality of wheat?
A.12.75
B.10.80
C.15.50
D.20.25
E.18.50 - How many kgs of rice of variety-1 costing Rs.42/kg should a shopkeeper mix with 25 kgs of rice of variety-2 costing Rs.24 per kg so that he makes a profit of 25% on selling the mixture at Rs.40/kg?
A.15
B.20
C.25
D.30
E.None of these - An alloy contains Brass, Iron and Zinc in the ratio 2:3:1 and another contains Iron, zinc and lead in the ratio 5:4:3.If equal weights of both alloys are melted together to form a third alloy, then what will be the weight of lead per kg in new alloy?
A.5 1/9
B.1/4
C.4 1/7
D.1/8
E.2/7 - A can contains 50 litres of milk. 10 litres of this milk is taken out and replaced with water. This process is repeated twice. Find the amount of remaining milk in the mixture?
A.127/3 litres
B.26 2/3 litres
C.87litres
D.128/5litres
E.27 2/3 litres - A milkman mixes 6 litres of free tap water with 20litres of pure milk. If the cost of pure milk is Rs.28 per litre the % Profit of the milkman when he sells all the mixture at the cost price is
A.25%
B.16.5%
C.30%
D.16(1/3)%
E.None of these - Rs 4000 is lent in 2 parts, 1 part at 8% per annum and 2nd part at 12% per annum. At the end of a year Rs 400 is received as simple interest. Find the part lent at 8% p.a.
A.3500
B.2500
C.2000
D.1500
E.1000 - A 144 litres of mixture contains milk and water in the ratio 5 : 7. How much milk need to be added to this mixture so that the new ratio is 23 : 21 respectively?
A.36 litres
B.40 litres
C.28 litres
D.32 litres
E.30 litres - In a 100 litre mixture of milk and water, the % of water is only 20%. The milkman gave 25 litres of this mixture to a customer and then added 25 litres of water to the remaining mixture. What is the % of milk in the final mixture?
A.68%
B.57%
C.60%
D.53%
E.None of these
- Two vessels A and B contain a mixture of Milk and Water. In the first vessel (i.e) Vessel A has the ratio of Milk to water is 8 : 3 and in the second vessel, Vessel B has the ratio of 5 : 1. A 35 litre capacity vessel is filled from these two vessels so as to contain a mixture of Milk and water in ratio of 4 : 1. Then how many litres should be taken from the first vessel, Vessel “A”.
A. 12 L
B. 17 L
C. 14 L
D. 11 L
E. None of the Above - When one litre of water is added to a mixture of milk and water, the new mixture contains 25% of milk. When one litre of milk is added to the new mixture, then the resulting mixture contains 40% milk. What is the percentage of milk in the original mixture?
A. 100/6 %
B. 50/6 %
C. 100/3 %
D. 50/3 %
E. None of the Above - The price of a box and a pen is Rs.60. The box was sold at a 40% profit and the pen at a loss of 10%. If the Shop keeper gains Rs.4 in the whole transaction, then how much is the cost price of Box?
A. Rs.10
B. Rs.30
C. Rs.20
D. Rs.40
E. None of the Above - A vessel contains a mixture of diesel and petrol in which there is 20% diesel. Five litres are drawn off and then the vessel is filled with petrol. If the diesel present in the mixture is now 15% then how much does the vessel hold?
A. 10 L
B. 20 L
C. 30 L
D. 40 L
E. None of the Above - In a lab, two chemical solutions Acid “A” with 90% purity and Acid “B” with 96% purity are mixed resulting in 24 litres of mixture of 92% purity. How much is the quantity of the first solution, Acid “A” in the resulting mixture?
A. 12 L
B. 20 L
C. 16 L
D. 14 L
E. None of the Above - 60 kg of a certain variety of Sugar at Rs.32 per kg is mixed with 48 kg of another variety of sugar and the mixture is sold at the average price of Rs.28 per kg. If there be no profit or no loss due to the new selling price, then what is the price of second variety of Sugar?
A. Rs.25
B. Rs.23
C. Rs.29
D. Rs.27
E. None of the Above - Six litre of milk was taken out from a vessel and is then filled with water. This operation is performed two more times. The ratio of the quantity of milk now left in vessel to that of the water is 8 : 27. How much is the quantity of the milk contained by the vessel originally?
A. 18 litre
B. 16 litre
C. 14 litre
D. 12 litre
E. None of the Above - A vessel is filled with 120 litres of Chemical solution, Acid “A”. Some quantity of Acid “A” was taken out and replaced with 23 litres of Acid “B” in such a way that the resultant ratio of the quantity of Acid “A” to Acid “B” is 4:1. Again 23 litres of the mixture was taken out and replaced with 28 litre of Acid “B”. What is the ratio of the Acid “A” to Acid “B” in the resultant mixture?
A. 43 : 29
B. 46 : 23
C. 47 : 21
D. 46 : 29
E. None of the Above - 18 litres of Petrol was added to a vessel containing 80 litres of Kerosene. 49 litres of the resultant mixture was taken out and some more quantity of petrol and kerosene was added to the vessel in the ratio 2:1. If the respective ratio of kerosene and petrol in the vessel was 4:1, what was the quantity of kerosene added in the vessel?
A. 1 litre
B. 2 litre
C. 5 litre
D. 3 litre
E. None of the Above - A vessel which contains a mixture of acid and water in ratio 13:4. 25.5 litres of mixture is taken out from the vessel and 2.5 litres of pure water and 5 litres of acid is added to the mixture. If resultant mixture contains 25% water, what was the initial quantity of mixture in the vessel before the replacement in litres?
A. 58 litre
B. 68 litre
C. 78 litre
D. 48 litre
E. None of the Above









