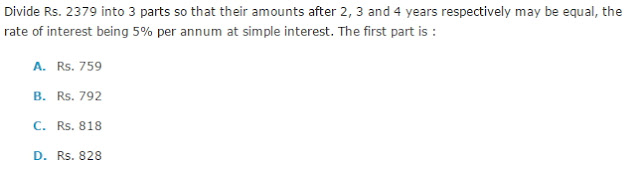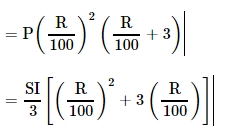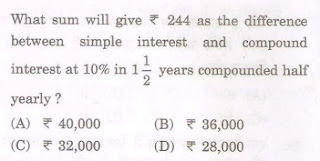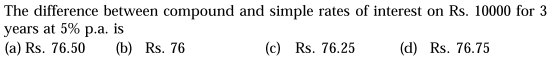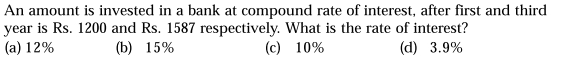Simple and Compound Interest Tricks – 2
Read this post carefully and note down all the formulas in a piece of paper for quick revision.
Q. 1) If a sum of money becomes 3 times itself in 20 years at simple interest. What is the rate of interest?
In such questions apply the direct formula-
Rate of interest = [100*(Multiple factor – 1)]/T
So R = 100*(3 – 1)/20
Answer : 10%
Note : With this formula you can find Rate if Time is given and Time if rate is given.
Q. 2)
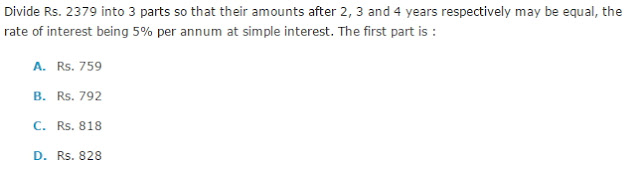
In such questions, just write this line :
1st part : 2nd part : 3rd part = 1/(100+T1 * r) : 1/(100+T2 * r) : 1/(100+T3 * r)
= 1/(100+2 * 5) : 1/(100+3 * 5) : 1/(100+4*5)
= 1/110 : 1/115 : 1/120
= 23*24 : 22*24 : 23*22
Hence 1st part = (23*24)/ (23*24 + 22*24 + 23*22) * 2379
Answer : 828
Note : Surprisingly, such questions when asked mostly have this same data, i.e., R=5% and T1, T2, T3 = 2, 3, 4 years, respectively. Only the Principal is changed. So it would be wise if you can just mug this line :
1st part : 2nd part : 3rd part = 23*24 : 22*24 : 23*22
Based on the above line, you would be able to solve such questions in a jiffy.
But note that it will only work if the question is on Simple Interest. Like the below question appeared in SSC CGL Tier 2-
Q. 4
Here the data is same. i.e., R=5% and T1, T2, T3 = 2, 3, 4 years, respectively. So we will write directly –
1st part : 2nd part : 3rd part = 23*24 : 22*24 : 23*22
A received = (23*24)/ (23*24 + 22*24 + 23*22) * 7930
Answer : Rs. 2760
Q. 5) If a certain sum of money P lent out for a certain time T amounts to P1 at R1% per annum and to P2 at R2% per annum, then
The above formula is for calculating the Time, if the question asks the rate, then just interchange the rate and time. Hence the formula will become
R = (P1 – P2)*100/P2T1 – P1T2
Apply the formula:
R = (650-600)*100/600*6 – 650*4
R = 5%
Alternative Method :
You can solve such questions quickly without mugging the above formula. How?
The sum amounts to Rs. 600 in 4 years and Rs. 650 in 6 years. This means the simple interest is Rs. 50 for 2 years (because the amount increased from Rs. 600 to Rs. 650 in 2 years)
So the SI for 4 years is Rs. 100 (we have seen earlier than SI is proportional. So if SI = 100 for 2 years, then SI = 150 for 3 years, SI = 250 for 5 years and so on)
Now SI = Rs. 100; P = 600-100 = Rs. 500; t = 4 years
R = 100*SI/(P*t) = 10000/2000
Answer: 5%
For CI, the formula is different
Difference between CI and SI
This topic is very important from examination point of view. Note the following things-
If t=1 year, then SI = CI
If t=2 years then difference between CI and SI can be given by two formulas-
If t=3 years then difference between CI and SI can be given by two formulas-
In all the above formulas we have assumed that the interest is compounded annually
Let us solve some CGL questions
Q. 6
A = P(1+r/100)^t
Given, A=1.44P t = 2 years
1.44P = P(1 + r/100)^2
r = 20%
Answer : (D)
Q. 7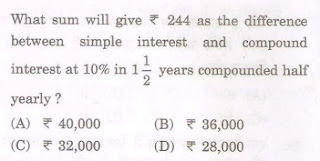
Here the interest is compounded half yearly, so the formulas we mugged earlier are of no use here. We will have to solve this question manually
SI = P*10*1.5/100 = 0.15P
CI = P(1 + 5/100)^3 – P = P(1.05^3 – 1)
Given CI – SI = 244
P(1.05^3 – 1) – 0.15P = 244
P = Rs. 32000
Answer : (C)
Q. 8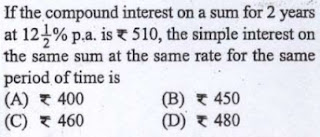
Time = 2 years
Hence apply the formula: Difference(D) = R*SI/200
CI – SI = R*SI/200
CI – SI = (12.5/200)*SI
510 = 1.0625*SI [Since CI = Rs. 510]
SI = Rs. 480
Answer : (D)
Q. 9
CI for 1st year = 10% of 1800 = Rs. 180
CI for 2nd years = 180 + 10% of 180 = Rs. 198
Total = 180+198 = Rs. 378
Hence time = 2 years
Or you can apply the formula
A = P(1+r/100)^t
Answer : (B)
Q. 10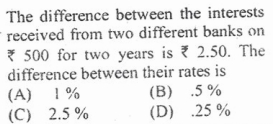
2.5 = P*R*2/100 – P*r*2/100
2.5 = 10R – 10r
R – r = 0.25
Answer : (D)
Q. 11
CI for 1st year = 5% of P = 0.05P
CI for 2nd year = 5% of P + 5% of (5% of P) = 0.05P + 0.0025P = 0.0525P
Total CI = 0.05P + 0.0525P = 0.1025P
Given, 0.1025P = 328
P = Rs. 3200
Answer : (C)
Note : You can solve this question by the formula A = P(1+r/100)^t as well
Q. 12
Note that in this question the CI for 2 years in not given, but the CI for the 2nd year is given.
CI for 2nd year = 10% of P + 10% of (10% of P) = 0.1P + 0.01P = 0.11P
Given, 0.11P = 132
P = Rs. 1200
Answer : (D)
Q. 13
Interest = Re. 1 per day = Rs. 365 for 1 year
SI = P*r*t/100
t=1, r=5%, SI = Rs. 365
So, P = 365*100/5 = Rs. 7300
Answer : (A)
Q. 14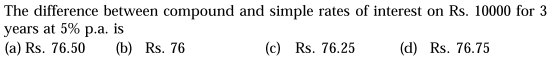
We know
Difference = P(r/100)^2(r/100 + 3)
P = Rs. 10000, r = 5%, t = 3 years
Hence D = Rs. 76.25
Answer : (C)
Q. 15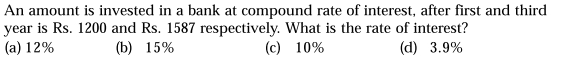
We know, R = [(y/x)^(1/T2 – T1) – 1]*100
= [(1587/1200)^1/(3 – 1) – 1]*100
= [(1587/1200)^1/2 – 1]*100
= 3/20 * 100
= 15%
Answer : (B)
So this is the end of CI and SI series. If you have any doubt in this topic, please drop a comment…